
With very fine pores one can make a fairly large volumes of spongy materials that can hold plenty of water without the weight of the water being enough to drain it. Spongy materials work the same way, by keeping small volumes of water in their pores. The thinner the tube, the taller the column of liquid that can be held by the tube. If you submerge the tube in water and you pull it out, the molecular forces between the water molecules and the tube will keep some water in the tube, even if it is open at the bottom. You can try this yourself with a narrow tube (I would say make it considerably smaller in inner diameter than a sixteenth of an inch). The point where this pressure law brakes down is when the surface tension of the water starts to play a leading role.

If the math does break down at some point. I want that to be true cost wise but can't wrap my head around it because it seems to fall apart in the extreme hypothetical case where at some point there just isn't enough water to exert 1000lbs. I ask this because the math says that structurally I can double the volume of the tank just by increasing the same materials in the width without any additional support required.
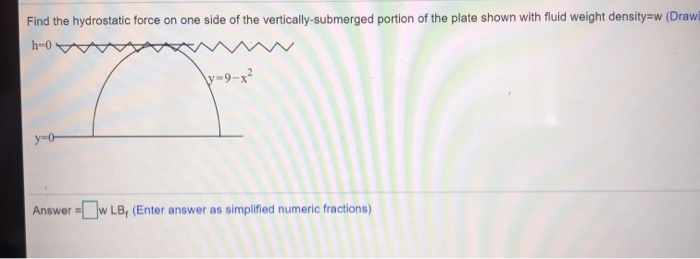
And if I theoretically continue to halve until the tank only holds 1 ounce of water the that 1 ounce still exerts 1000 lbs on the long side. Same formula states that since only depth matters in pressure then if I halve the width of the tank (front to back) then the total force on the long side remains the same 1000 lbs. 43 is psi per foot of depth and average psi is at depth 1 foot in this case. I building a tank for water 2ft high, 2ft wide (front to back), 8 feet long (left to right) Common formulas dictate the total force on the long sides is approx 1000lbs (w x h x.


 0 kommentar(er)
0 kommentar(er)
